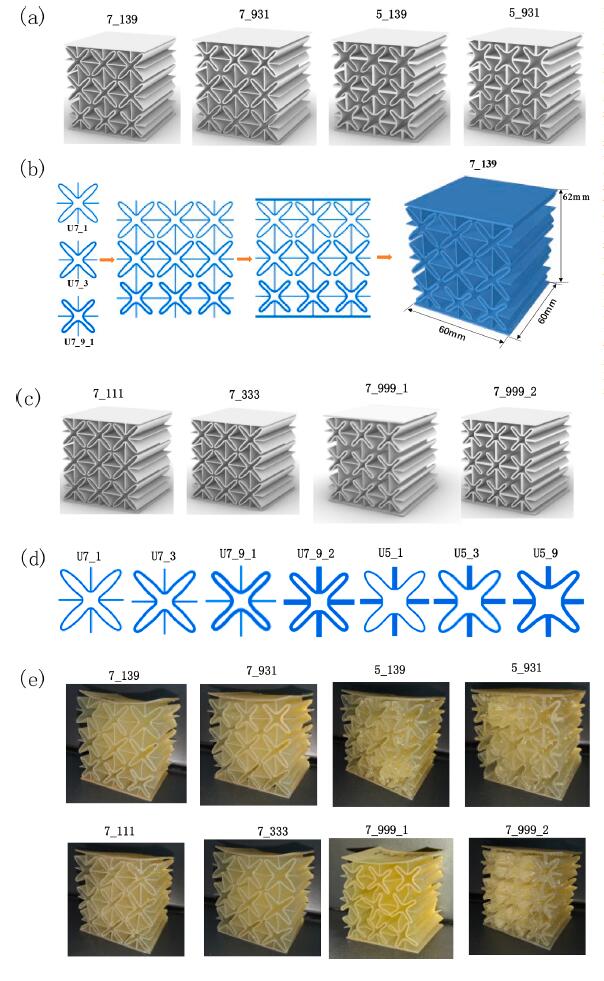
Deep learning-enhanced design for functionally graded auxetic lattices
Auxetic materials with counterintuitive negative Poisson’s ratio have been of significant interest due to potential
applications across diverse engineering fields. Functionally grading such auxetics further enables customization
of the structural response and harnesses the potential for multi-functional applications. However, a critical
challenge in designing functionally graded lattices is to efficiently determine the spatial variation of the functional gradient and the corresponding geometric designs to achieve the desired response. In this paper, a highly efficient deep learning-based inverse design framework for functionally graded tetra-petal auxetics with spatially tailored properties is presented. This framework significantly improves the efficiency of tailoring functionally graded auxetics where many unit cells need to be tailor-designed. The graded tetra-petal auxetics obtained from the inverse design framework are additively manufactured and subjected to impact tests. The results show superior impact performance compared with uniform designs, demonstrating the effectiveness of the proposed inverse design framework, which can be inspirable to promote advanced structures/materials with enhanced impact resistance.